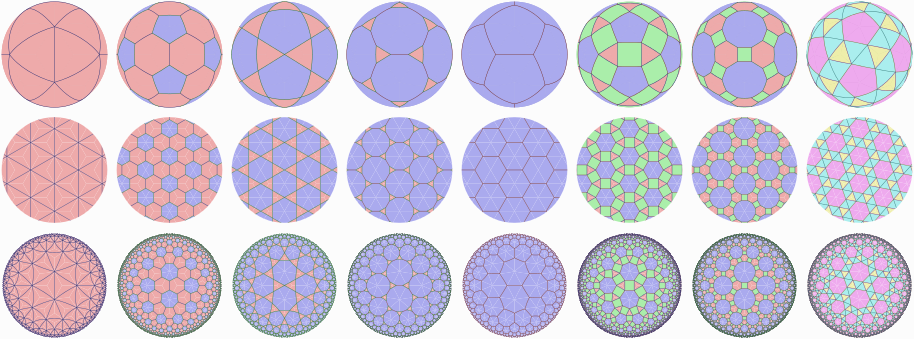
Welcome to my visualization of uniform tilings by Wythoffian construction, so named after mathematician Willem Abraham Wythoff. A uniform tiling is a tessellation of a two-dimensional surface by regular polygon faces with the restriction of being vertex-transitive. Such tilings are demonstrated in spherical, Euclidean and hyperbolic geometries.
The tiling is constructed by your web browser and allows some interactiveity. A recent web browser is required for this to work correctly. Please note the hyperbolic tilings can take some time to compute. If a box appears asking if you want to stop the script then just let it continue and all should be fine. Click the link below to get going!
It's an on going project where I'm adding features over time, so please check back often. The last update was 2015-01-04.
The spherical tilings of the top row are based on the (3 5 2) fundamental. The Euclidean tilings of the middle row are based on the (3 6 2) fundamental. The hyperbolic tilings of the bottom row are based on the (3 7 2) fundamental.

Each row presents the following sequence of Wythoffian operations: primal, truncation, rectification, bitruncation, birectification, cantellation, omnitruncation, snub.
Many pretty tilings (although rarely uniform) can be made by changing the location of the generator point and playing with the display options. Here are some examples:
Tiling information from Wikipedia:
The following sites have been a great source and inspiration:
Sam Gratrix, Gratrix.net.